
The clever graphic is one of Art Carden’s economic “memes” that has been circulating around in cybersphere for a day or two. The lesson, of course, is the law of demand — price goes up, people buy less; price goes down, people buy more.
But Slate’s Matthew Yglesias steps up and says that maybe it isn’t so!
Imagine a world with two goods—beer and bourbon—such that beer is cheaper per unit of alcohol than bourbon, but bourbon is tastier. Drinkers arrive at some kind of beer/bourbon mix based on their desires to (a) get drunk, (b) drink something tasty, and (c) have money left over for other activities. Now the price of beer falls…
The first thing to notice is that this is not a two-good world, as assumption (c) places us squarely in a three-good world: beer, bourbon, and other activities. More on that in a minute. Yglesias runs through a couple possibilities where people indeed drink more beer when the price decreases, as one would expect, but then he finishes with this zinger:
Yet another possibility is that people hold total spending and total alcohol consumption (constant) and use the budgetary headroom opened up by cheaper beer to buy less beer and extra bourbon.
So, let’s break this down. First off, total spending here is simply another way of saying the consumer has an income constraint. Second, the “budgetary headroom opened up” is the income effect from the price of a good in the consumption set decreasing. In other words, when the price of a good in a consumer’s consumption set falls, he effectively becomes richer because he can continue to buy his current consumption set, but now he has money left over to buy more beer, more bourbon, or more other activities.
If we go ahead and assume that our consumer has convex preferences, our standard assumption, then we know that holding utility constant that when the price of beer falls, the substitution effect will indeed induce more beer consumption.
But what about the income effect from the budgetary headroom? It’s possible that greater income leads to lower consumption of some goods (Ramen Noodles, bus rides, World Series viewings), and we call such goods inferior goods. Anyone who has taken price theory knows that I don’t need three goods to get people to buy more bourbon and less beer — I simply need beer to be an inferior good that is subject to a really big income effect. That is, the price of beer falls, the substitution effect leads our consumer to buy more beer, but the income effect from greater budgetary headroom overwhelms the substitution effect. Beer is a Giffen good and we call it a day.
Of course, economists have been looking for Giffen goods for a very long time, and there isn’t a long list, so maybe that isn’t the best route to go.
Instead of this, Yglesias provides this additional “iso-alcohol” constraint, where consumers want to keep overall alcohol consumption constant. If the standard two-good model, if you hand someone $1000, the beneficiary buys more beer and less bourbon (if bourbon is inferior), more bourbon and less beer (if beer is inferior), or more of both (if both are normal). With the iso-alcohol constraint, however, the third case is off the table and additional money will go to some other activity. Hence, for Yglesias’ case of less beer with more “budgetary headroom” to hold, we know that we would have to be in the second case with beer being an inferior good. At the same time, though, if I hand someone $1000, it’s possible that they buy more bourbon and less beer, which is Yglesias’ point to begin with: “use the budgetary headroom opened up by cheaper beer to buy less beer and extra bourbon.”
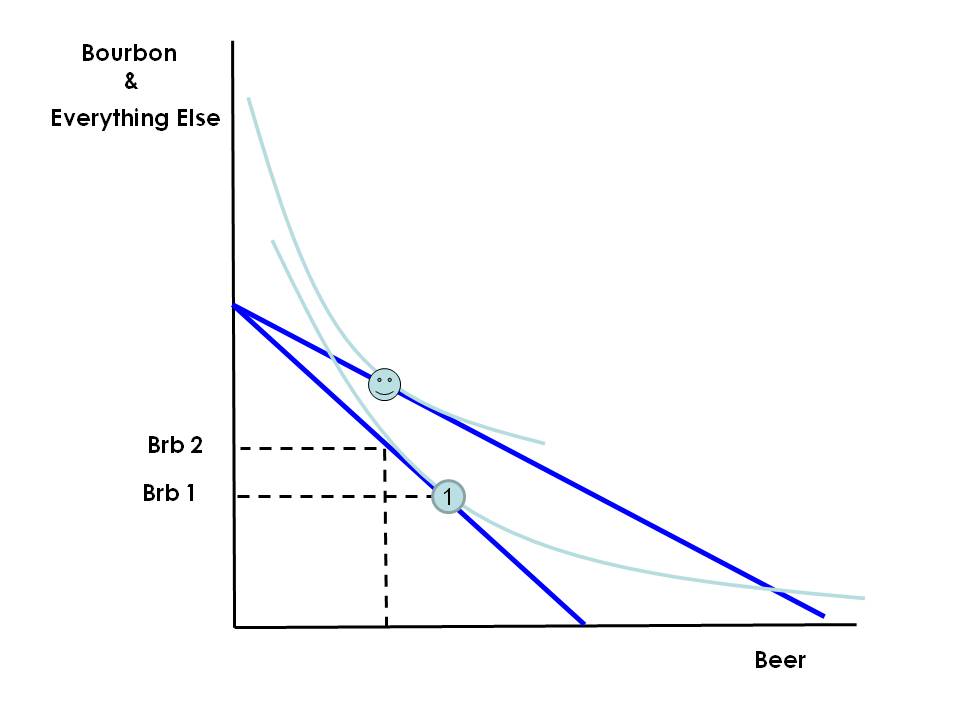
So, let’s collapse this back down to a world where we have two goods: (1) beer and (2) bourbon and everything else, where the consumer is subject to both the income and the iso-alcohol constraint (linear combinations of beer and bourbon that lead to an equivalent level of insobriety). For visual simplicity, assume that initial income constraint is the same as the initial iso-alcohol constraint. That is, the relative price of beer and bourbon is the same as the relative weights needed to achieve some level of insobriety. Also for simplicity, assume that the consumer initially spends all his income on beer and bourbon. The optimal consumption set denoted by the circle with the 1 with bourbon consumption of Brb 1, where Brb 1 constitutes all spending on goods other than beer.
Now along comes the decrease in the price of beer and all of a sudden we have a new budget constraint, one that pivots outward with the same Y intercept but a new X intercept. The potential consumption set has expanded. Clearly, the consumer can buy more than he bought before, and a new optimum consistent with less beer consumption is illustrated by the smiley.
Because the consumer wants to maintain the same level of alcohol consumption, the amount spent on bourbon has to be on the original budget constraint — in this case the distance Brb 2 represents the amount spent on bourbon and the amount necessary to maintain that level of alcohol consumption. The distance between Brb 2 and the smiley is the amount spent on other stuff. As you can see, Yglesias’ possibility collapses to the observation that “beer could be a Giffin good.” In other words, not likely at all.
It’s probably worth noting that the iso-alcohol constraint is problematic in its own right, as it implies a minimum level of consumption that might not be viable in a world where the price of beer and bourbon go up, or income decreases. In our example if the consumer is initially exhausting his income on beer and bourbon, any price increase or income decrease has the unhappy result that the iso-alcohol constraint cannot be satisfied.
Thank you to loyal reader Mr. P for the tip.